Сфероид

Сфероиды с вертикальными осями вращения
сплюснутый		вытянутый

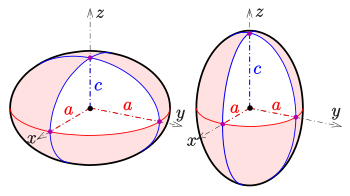
Сфероида , также известный как эллипсоид вращения или вращение эллипсоида , является квадрикой поверхности получается вращающейся собой эллипс об одном из его главных осей; другими словами, эллипсоид с двумя равными полудиаметрами . Сфероид имеет круговую симметрию .

Если эллипс вращается вокруг своей главной оси, в результате получается вытянутый (удлиненный) сфероид, по форме напоминающий мяч для американского футбола или регби . Если эллипс вращается вокруг своей малой оси, в результате получается сплющенный (сплющенный) сфероид в форме чечевицы или простого M&M . Если образующий эллипс - круг, результатом будет сфера .

Благодаря совместному действию силы тяжести и вращения , то фигура Земли (и все планеты ) не совсем сфера, но вместо этого слегка уплощенной в направлении его оси вращения. По этой причине в картографии и геодезии Землю часто аппроксимируют сплюснутым сфероидом, известным как опорный эллипсоид , а не сферой. Текущая модель Мировой геодезической системы использует сфероид, радиус которого составляет 6 378,137 км (3 963,191 миль) на экваторе и 6 356,752 км (3 949,903 миль) на полюсах .

Слово сфероид первоначально означало «приблизительно сферическое тело», допускающее неровности даже за пределами двух- или трехосной эллипсоидальной формы; именно так этот термин используется в некоторых старых работах по геодезии (например, имея в виду усеченные сферические гармонические разложения модели геопотенциала силы тяжести Земли ). ^[1]

Уравнение [ править ]

Назначение полуосей на сфероиде. Это сплюснутая , если

с <

(слева) и вытянутым , если

с >

(справа).

Уравнение трехосного эллипсоида с центром в начале координат с полуосями $a$ , $b$ и $c,$ выровненными вдоль осей координат, имеет вид

{\frac {x^{2}}{a^{2}}}+{\frac {y^{2}}{b^{2}}}+{\frac {z^{2}}{c^{2}}}=1.

Уравнение сфероида с $z$ в качестве оси симметрии задается положением $a = b$ :

{\frac {x^{2}+y^{2}}{a^{2}}}+{\frac {z^{2}}{c^{2}}}=1.

Полуось $a$ - это экваториальный радиус сфероида, а $c$ - расстояние от центра до полюса вдоль оси симметрии. Возможны два случая:

$c < a$ : сплюснутый сфероид
$c > a$ : вытянутый сфероид

Случай $a = c$ сводится к сфере.

Свойства [ править ]

Площадь [ править ]

Сплюснутый сфероид с $c < a$ имеет площадь поверхности

S_{\rm {oblate}}=2\pi a^{2}\left(1+{\frac {1-e^{2}}{e}}{\text{arctanh}}\,e\right)=2\pi a^{2}+\pi {\frac {c^{2}}{e}}\ln \left({\frac {1+e}{1-e}}\right)\quad {\mbox{where}}\quad e^{2}=1-{\frac {c^{2}}{a^{2}}}.

Сплюснутый сфероид создается вращением вокруг оси $z$ эллипса с большой полуосью $а$ и малой полуосью $с$ , поэтому $е$ можно определить как эксцентриситет . (См. Эллипс .) ^[2]

Вытянутый сфероид с $c > a$ имеет площадь поверхности

S_{\rm {prolate}}=2\pi a^{2}\left(1+{\frac {c}{ae}}\arcsin \,e\right)\qquad {\mbox{where}}\qquad e^{2}=1-{\frac {a^{2}}{c^{2}}}.

Вытянутый сфероид образуется вращением вокруг оси $z$ эллипса с большой полуосью $с$ и малой полуосью $а$ ; поэтому $e$ можно снова определить как эксцентриситет . (См. Эллипс .) ^[3]

Эти формулы идентичны в том смысле, что формулу для $S- сплющенного$ сфероида можно использовать для расчета площади поверхности вытянутого сфероида и наоборот. Однако тогда $e$ становится мнимым и больше не может быть напрямую отождествлено с эксцентриситетом. Оба эти результата могут быть представлены во многих других формах, используя стандартные математические тождества и отношения между параметрами эллипса.

Объем [ править ]

Объем внутри сфероида (любого вида) равен . Если - экваториальный диаметр, а - полярный диаметр, объем равен . ${\frac {4\pi }{3}}a^{2}c\approx 4.19a^{2}c$ $A=2a$ $C=2c$ ${\frac {\pi }{6}}A^{2}C\approx 0.523A^{2}C$

Кривизна [ править ]

If a spheroid is parameterized as

{\vec {\sigma }}(\beta ,\lambda )=(a\cos \beta \cos \lambda ,a\cos \beta \sin \lambda ,c\sin \beta );\,\!

where $β$ is the reduced or parametric latitude, $λ$ is the longitude, and $- π / 2 < β < + π / 2$ and $-π < λ < +π$ , then its Gaussian curvature is

K(\beta ,\lambda )={c^{2} \over \left(a^{2}+\left(c^{2}-a^{2}\right)\cos ^{2}\beta \right)^{2}};\,\!

and its mean curvature is

H(\beta ,\lambda )={c\left(2a^{2}+\left(c^{2}-a^{2}\right)\cos ^{2}\beta \right) \over 2a\left(a^{2}+\left(c^{2}-a^{2}\right)\cos ^{2}\beta \right)^{\frac {3}{2}}}.\,\!

Both of these curvatures are always positive, so that every point on a spheroid is elliptic.

Aspect ratio[edit]

The aspect ratio of an oblate spheroid/ellipse, $c : a$ , is the ratio of the polar to equatorial lengths, while the flattening (also called oblateness) $f$ , is the ratio of the equatorial-polar length difference to the equatorial length:

f={\frac {a-c}{a}}=1-{\frac {c}{a}}.

The first eccentricity (usually simply eccentricity, as above) is often used instead of flattening.^[4] It is defined by:

e={\sqrt {1-{\frac {c^{2}}{a^{2}}}}}

The relations between eccentricity and flattening are:

e={\sqrt {2f-f^{2}}}

,

f=1-{\sqrt {1-e^{2}}}

All modern geodetic ellipsoids are defined by the semi-major axis plus either the semi-minor axis (giving the aspect ratio), the flattening, or the first eccentricity. While these definitions are mathematically interchangeable, real-world calculations must lose some precision. To avoid confusion, an ellipsoidal definition considers its own values to be exact in the form it gives.

Applications[edit]

The most common shapes for the density distribution of protons and neutrons in an atomic nucleus are spherical, prolate, and oblate spheroidal, where the polar axis is assumed to be the spin axis (or direction of the spin angular momentum vector). Deformed nuclear shapes occur as a result of the competition between electromagnetic repulsion between protons, surface tension and quantum shell effects.

Oblate spheroids[edit]

The planet Jupiter is an oblate spheroid with a flattening of 0.06487

The oblate spheroid is the approximate shape of rotating planets and other celestial bodies, including Earth, Saturn, Jupiter, and the quickly spinning star Altair. Saturn is the most oblate planet in the Solar System, with a flattening of 0.09796. See planetary flattening and equatorial bulge for details.

Enlightenment scientist Isaac Newton, working from Jean Richer's pendulum experiments and Christiaan Huygens's theories for their interpretation, reasoned that Jupiter and Earth are oblate spheroids owing to their centrifugal force.^[5]^[6] Earth's diverse cartographic and geodetic systems are based on reference ellipsoids, all of which are oblate.

A science-fiction example of an extremely oblate planet is Mesklin from Hal Clement's novel Mission of Gravity.

Prolate spheroids[edit]

A rugby ball.

The prolate spheroid is the approximate shape of the ball in several sports, such as in the rugby ball.

Several moons of the Solar System approximate prolate spheroids in shape, though they are actually triaxial ellipsoids. Examples are Saturn's satellites Mimas, Enceladus, and Tethys and Uranus' satellite Miranda.

In contrast to being distorted into oblate spheroids via rapid rotation, celestial objects distort slightly into prolate spheroids via tidal forces when they orbit a massive body in a close orbit. The most extreme example is Jupiter's moon Io, which becomes slightly more or less prolate in its orbit due to a slight eccentricity, causing intense volcanism. The major axis of the prolate spheroid does not run through the satellite's poles in this case, but through the two points on its equator directly facing toward and away from the primary.

The term is also used to describe the shape of some nebulae such as the Crab Nebula.^[7] Fresnel zones, used to analyze wave propagation and interference in space, are a series of concentric prolate spheroids with principal axes aligned along the direct line-of-sight between a transmitter and a receiver.

The atomic nuclei of the actinide and lanthanide elements are shaped like prolate spheroids.^[8] In anatomy, near-spheroid organs such as testis may be measured by their long and short axes.^[9]

Many submarines have a shape which can be described as prolate spheroid.^[10]

Dynamical properties[edit]

For a spheroid having uniform density, the moment of inertia is that of an ellipsoid with an additional axis of symmetry. Given a description of a spheroid as having a major axis $c$ , and minor axes $a$ and $b$ , the moments of inertia along these principal axes are $C$ , $A$ , and $B$ . However, in a spheroid the minor axes are symmetrical. Therefore, our inertial terms along the major axes are:^[11]

A=B={\frac {1}{5}}M(a^{2}+c^{2}),

C={\frac {1}{5}}M(a^{2}+a^{2})={\frac {2}{5}}M(a^{2}),

where $M$ is the mass of the body defined as

M={\frac {4}{3}}\pi \rho ca^{2}.

References[edit]

^ Torge, Wolfgang (2001). Geodesy (3rd ed.). Walter de Gruyter. p. 104. ISBN 9783110170726.
^ A derivation of this result may be found at "Oblate Spheroid - from Wolfram MathWorld". Mathworld.wolfram.com. Retrieved 24 June 2014.
^ A derivation of this result may be found at "Prolate Spheroid - from Wolfram MathWorld". Mathworld.wolfram.com. 7 October 2003. Retrieved 24 June 2014.
^ Brial P., Shaalan C.(2009), Introduction à la Géodésie et au geopositionnement par satellites, p.8
^ Greenburg, John L. (1995). "Isaac Newton and the Problem of the Earth's Shape". History of Exact Sciences. Springer. 49 (4): 371–391. doi:10.1007/BF00374704. JSTOR 41134011. S2CID 121268606.
^ Durant, Will; Durant, Ariel (28 July 1997). The Story of Civilization: The Age of Louis XIV. MJF Books. ISBN 1567310192.
^ Trimble, Virginia Louise (October 1973), "The Distance to the Crab Nebula and NP 0532", Publications of the Astronomical Society of the Pacific, 85 (507): 579, Bibcode:1973PASP...85..579T, doi:10.1086/129507
^ "Nuclear fission - Fission theory". Encyclopedia Britannica.
^ Page 559 in: John Pellerito, Joseph F Polak (2012). Introduction to Vascular Ultrasonography (6 ed.). Elsevier Health Sciences. ISBN 9781455737666.
^ "What Do a Submarine, a Rocket and a Football Have in Common?". Scientific American. 8 November 2010. Retrieved 13 June 2015.
^ Weisstein, Eric W. "Spheroid". MathWorld--A Wolfram Web Resource. Retrieved 16 May 2018.

[1] Torge, Wolfgang (2001). Geodesy (3rd ed.). Walter de Gruyter. p. 104. ISBN 9783110170726.

[2] A derivation of this result may be found at "Oblate Spheroid - from Wolfram MathWorld". Mathworld.wolfram.com. Retrieved 24 June 2014.

[3] A derivation of this result may be found at "Prolate Spheroid - from Wolfram MathWorld". Mathworld.wolfram.com. 7 October 2003. Retrieved 24 June 2014.

[4] Brial P., Shaalan C.(2009), Introduction à la Géodésie et au geopositionnement par satellites, p.8

[5] Greenburg, John L. (1995). "Isaac Newton and the Problem of the Earth's Shape". History of Exact Sciences. Springer. 49 (4): 371–391. doi:10.1007/BF00374704. JSTOR 41134011. S2CID 121268606.

[6] Durant, Will; Durant, Ariel (28 July 1997). The Story of Civilization: The Age of Louis XIV. MJF Books. ISBN 1567310192.

[Trimble1973-7] Trimble, Virginia Louise (October 1973), "The Distance to the Crab Nebula and NP 0532", Publications of the Astronomical Society of the Pacific, 85 (507): 579, Bibcode:1973PASP...85..579T, doi:10.1086/129507

[8] "Nuclear fission - Fission theory". Encyclopedia Britannica.

[9] Page 559 in: John Pellerito, Joseph F Polak (2012). Introduction to Vascular Ultrasonography (6 ed.). Elsevier Health Sciences. ISBN 9781455737666.

[scientific_american-10] "What Do a Submarine, a Rocket and a Football Have in Common?". Scientific American. 8 November 2010. Retrieved 13 June 2015.

[11] Weisstein, Eric W. "Spheroid". MathWorld--A Wolfram Web Resource. Retrieved 16 May 2018.

[1]